Imaginary Numbers: A number whose square is negative, is known as an imaginary number, e.g. : \(\sqrt{-1}, \sqrt{-2}\), etc.
\(\sqrt{-1}\) is denoted by the Greek letter i (iota).

Powers of i : i¹ = – 1; i²= – 1; i³ = — i, i⁴ = 1, etc.

An Important Result : For any two real numbers a and b,
\(\sqrt{a} \times \sqrt{b}=\sqrt{a b}\) is true only when at least one of a and b is either 0 or positive.
In fact, \(\sqrt{-a} \times \sqrt{-b}=(i \sqrt{a})(i \sqrt{b})=i^{2} \sqrt{a b}=-\sqrt{a b}\), where a and b are positive real numbers.

Complex Number : Any number of the form x + iy, where x, y are real numbers and i \(\sqrt{-1}\) is called a complex number.

It is denoted by z, i.e., z = x + iy. The real and imaginary parts of a complex number z are denoted by Re(z) and Im(z) respectively. Thus, if z = x + iy, then Re(z) = x and Im(z) = y.

Note : Every real number is a complex number, for if a e R, it can be written as a – a + i0.

Purely Real and Purely Imaginary Numbers : A complex number z is said to be
(i) purely real, if Im(z) = 0.
(ii) purely imaginary, if Re(z) = 0.

Conjugate of a Complex Number : The conjugate of a complex number z, denoted by \(\bar{z}\) is the complex number obtained by changing the sign of imaginary part of z i.e., if z = a + ib, then \(\bar{z}\) = a-ib.

Modulus of a Complex Number: The modulus of a complex number, z- a + ib, denoted by |z |, is defined as |z | = \(\sqrt{x^{2}+y^{2}}\)

Sum, Difference and Product of Complex Numbers : For any complex numbers z₁ = a + ib and z₂ = c + id, it is defined as
(i) Z₁ + z₂ = (a + ib) + (c + id) = (a + c) + i(b + d).
(ii) z₁ – z₂ = (a + ib) – (c + id) = (a -c) + i(b – d).
(iii) z₁z₂ = (a + ib)(c + id) = (ac -bd) + i(ad – bc).

Properties of Complex Numbers:

1. A real number can never be equal to an imaginary number.
2. (a + ib) = 0 ⇔ a = 0 and 6 = 0.
3. Two complex numbers are equal only when their real and imaginary parts are separately equal.
i.e., if a + ib = c + id, then a = c and b = d.

4. If z is a complex number, then :
(i) (\(\bar{z}\)) = z
(ii) (z + \(\bar{z}\)) is real.
(iii) (z – \(\bar{z}\)) is zero or an imaginary number.
(iv) z + \(\bar{z}\) = 2Re(z) and (z – \(\bar{z}\)) = 2iIm(z).
(v) z\(\bar{z}\) – |z |² and, therefore, z\(\bar{z}\) is real.

5. If z₁ and z₂ are complex numbers, then :
(i) \(\left(\overline{z_{1}+z_{2}}\right)=\overline{z_{1}}+\overline{z_{2}}\)
(ii) \(\left(\overline{z_{1}-z_{2}}\right)=\overline{z_{1}}-\overline{z_{2}}\)
(iii) \(\left(\overline{z_{1} z_{2}}\right)=\overline{z_{1}} \cdot \overline{z_{2}}\)
(iv) \(\left|z_{1} z_{2}\right|=\left|z_{1}\right|\left|z_{2}\right|\)

6. For any complex number z, |z | = 0 ⇔ z = 0.
7. The sum and product of two complex numbers are real if and only if they are conjugate of each other.
8. The conjugate and the reciprocal of a non-zero complex number z are equal if and only if | z | = 1.
9. Reciprocal or multiplicative inverse of a + ib
= \(\frac{1}{a+i b}=\frac{1}{a+i b} \times \frac{a-i b}{a-i b}=\frac{a}{a^{2}+b^{2}}-i\left(\frac{b}{a^{2}+b^{2}}\right)\)

10. For any integer k,
(i) i^2k = (-1)^k , i^{2k+ 1} = (- 1)^ki
(ii) i^4k = (i⁴)^k = 1, i^{4k+ 1} = i^4k+2 = i² = -1,
i^4k+3 = i³ = i² x i = -i

Geometrical Representation of a Complex Number : To every complex number z = x + iy, there is one and only one point P(x, y) in the plane, and conversely to every point (x,y) in the plane, there is one and only one complex number z = x + iy.

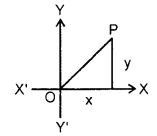
The plane, representing the complex numbers, as an ordered pair of real numbers is called the Complex plane or Gaussian plane or Argand plane.

Modulus and Amplitude of a Complex Number : Let a poin^ P represents a complex number z = x + iy in the complex plane. Then, the length OP is called the modulus of the complex number z and is denoted by |z|.

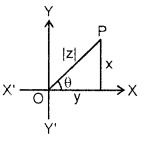
∴ |z| = OP = |x + iy|
= \(\sqrt{x^{2}+y^{2}}\) .

The angle XOP = θ is called the amplitude or argument of 2 and is written as amp. z or arg. z. The value of θ satisfying – π < θ < π is called the principal value of amp z.

Polar Form of a Complex Number : Let z – a + ib be represented by P(a, b).
Let OP = r, ∠XOP = θ.
Then, a – rcos θ,
b = rsinθ
= r² = a² + b²
⇒ r = |2|
and tanθ = \(\frac{b}{a}\)
⇒ cosθ = \(\frac{a}{r}\) ,sinθ = \(\frac{b}{r}\).
The form 2 = r(cos θ + isin θ) is called the Polar Form.

Polynomial Equation : A polynomial equation of a degree x has n roots.

Solutions of Quadratic Equation: The solutions of quadratic equation
ax² + bx + c = 0,
where a, b, c ∈ R, a ≠ 0, b2² – 4ac < 0 are given by
x = \(\frac{-b \pm\left(\sqrt{4 a c-b^{2}}\right) i}{2 i}\)

D = b² – 4ac is called the discriminant of the quadratic equation.