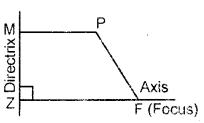
Conic : Let T be a fixed line and F be a fixed point (not on line T).
Let P be any point in the plane of the line T and the point F. Then, the set of all points P, such that | FP | = e | PM |, where M is the foot’ of perpendicular from P on the line l and e > 0 is . a fixed real number is called a conic.

Notes :

1. The fixed point F is called focus of the conic and the fixed line T is called directrix of the conic associated with F.
2. The positive real number e is called eccentricity of the conic.
3. A line through the focus F and perpendicular to the directrix l is called axis of the conic.
4. The point of intersection of the conic and its axis is called
   vertex.
5. A conic with eccentricity ‘e’ is called
   (i) a parabola, if e = 1. (ii) an ellipse, if e < 1. (iii) a hyperbola if e > 1..

Parabola : Let T be a fixed line and F be a fixed point (not on 1). Let P be any point in the plane of line l and the point F. Let M be the foot of perpendicular from P on the line l. Then, the set of all points P such that | FP | = | PM | is called a parabola.

Equation of the Parabola (Standard Form): The equation of the parabola in the standard form is y² = 4ax.

Latus Rectum : The latus rectum of conic is the chord passing through the focus and perpendicular to its axis.

Length of Latus Rectum : The length of the latus rectum of the parabola y² = 4ax is 4a.

Focal Distance : The distance of any point on parabola from the focus is called focal distance of that point.

Focal Chord : Any chord that passes through the focus of the parabola is called a focal chord of the parabola.

The focal distance of a point P(x₁, y₁ of the parabola y² = 4ax is a + x₁

Main Facts about Four Standard Forms of Parabola

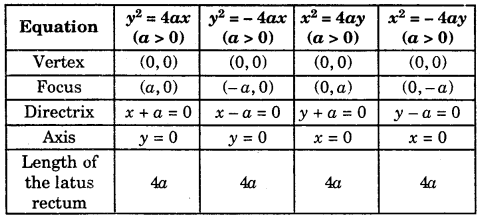
Ellipse : Let l be a fixed line, F be a fixed point (not on l). Let P be any point in the plane of the line l and the point F.

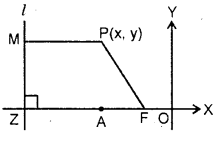
Let M be the foot of perpendicular from P on the line l.
Then, the set of all points P such that | FP | = e | PM | (0 < e < 1) is called an ellipse.

Notes : 1. The fixed line is called directrix of the ellipse.
2. The fixed point F is called focus of the ellipse.
3. The positive real number e < 1 is called eccentricity of the ellipse.

Standard form of an Ellipse : The equation of the ellipse in the standard form is \(\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}\) = 1, where b² = a²( 1 – e²) = a² – a²e².
Putting ae = c. Therefore, c² = a² – b².

Note: The ellipse \(\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}\) = 1 has two foci, namely (ae,o) and (- ae, 0) and their respective directrices are x – \(\frac{a}{e}\) = 0 and x + \(\frac{a}{e}\) = 0.

Main Facts about two Standard forms of Ellipse

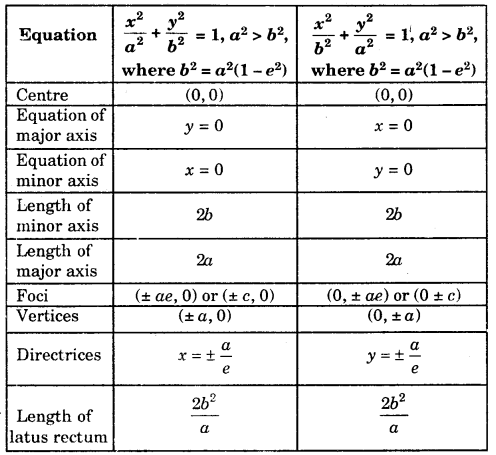
Notes : 1. The focal distances of any point (x₁, y₁) of the ellipse \(\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}\) = 1 are a ± ex₁
2. Sum of the focal distances of a point of the ellipse is equal to length of the major axis.

Hyperbola: Let l be a fixed line and F be a fixed point (not on l). Let P be any point in the plane of the line l and the point F. Let M be the foot of perpendicular from P on the line l. Then, the set of all points P such that | FP | = e | PM | (e > 1) is called a hyperbola.

2. Sum of the focal distances of a length of the major axis.

Hyperbola: Let l be a fixed line and F be a fixed point (not on l). Let P be any point in the plane of the line l and the point F. Let M be the foot of perpendicular from P on the line l. Then, the set of all points P such that | FP | = e | PM | (e > 1) is called a hyperbola.

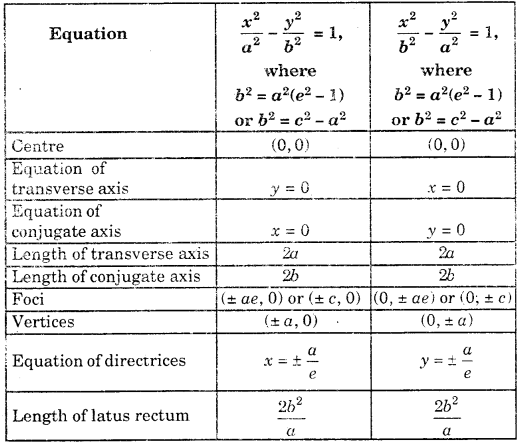
Standard form of Hyperbola : The equation of a hyperbola in the standard form is \(\frac{x^{2}}{a^{2}}-\frac{y^{2}}{b^{2}}\) = 1, where b² = a²(e² – 1). Putting ae -c, b² – c² – a² or c² – a² + b².

Notes: 1. The hyperbola \(\frac{x^{2}}{a^{2}}-\frac{y^{2}}{b^{2}}\) = 1 has two foci namely (- ae, 0) and (ae, 0) with two respective directrices as x + \(\frac{a}{e}\) = 0 and x – \(-\frac{a}{e}\) = 0

2. | ex₁ – a | and | ex₁ + a | are the focal distances of any point (x₁, y₁) on the hyperbola \(\frac{x^{2}}{a^{2}}-\frac{y^{2}}{b^{2}}\) = 1.

Main facts about two standard forms of hyperbola

Circle : The locus of a point which moves in a plane such that its distance from a fixed point, in the plane, is always a constant, is called a circle. The fixed point is called the centre and the constant distance is called the Radius of the circle.

Equation of the Circle : The equation of a circle with centre (h, k) and radius V’ is given by (x – h)² + (y – k)² = r².
If the centre of a circle is origin and radius is ‘r’ then its equation is x² + y² = r².

General Equation of a Circle : The general equation of a circle is x² + y² + 2gx + 2fy + c = 0.

Its centre is (-g,-f) and radius = \(\sqrt{g^{2}+f^{2}-c}\)

Note : 1. If g² + f² – c = 0, then radius = 0.
In this case, x² +y² + 2gx + 2fy + c = 0 represents a circle whose radius is zero. Such a circle is called a Point Circle, [i.e., it represents the point (-g, -f)].

2. If g² + f² – c < 0, then radius is non-real. In this case, x2 +y2 + 2gx + 2fy + c = 0 represents the empty set. 3. If g2 + f2 – c > 0, then radius is real and therefore,
x² + y² + 2gx + 2fy + c = 0 represents a circle whose centre is at the point (- g,- f) and radius = \(\sqrt{g^{2}+f^{2}-c}\). (where g² + f² – c > 0).

Conditions for an Equation to Represent a Circle :

(i) It should be a second degree equation in x and y.
(ii) Coefficient of x² = Coefficient of y² ≠ 0.
(iii) It should not contain any term of the product xy.

Method to find the centre, radius of a circle :

(i) Write the equation of the circle with the coefficient of x² and y² being unity.
(ii) Compare this with x² + y² + 2gx + 2fy + c = 0 and find the value of g,f and c.
(iii) Then, (-g,-f) is the centre and \(\sqrt{g^{2}+f^{2}-c}\) is the radius of the circle.